Deviations from lithostatic pressure: Fact of Fiction? This question has been a “hot-topic” in the geology community especially in the last 15 years. It is also a title of the special issue in Journal of Metamorphic Geology, which Lucie Tajcmanova edited.
Geologists commonly assume that pressures obtained from pressure-temperature (P-T) estimates of metamorphic assemblages are equal to lithostatic pressures. The lithostatic pressure is the pressure resulting only from the mass of the overburden rock and is equivalent to the hydrostatic pressure in fluids at rest. The lithostatic assumption is a simplification that can, to a certain degree, restrict our understanding of lithospheric processes. However, it is convenient because pressure can then be directly converted into a burial depth by assuming typical densities for crustal and mantle rocks.
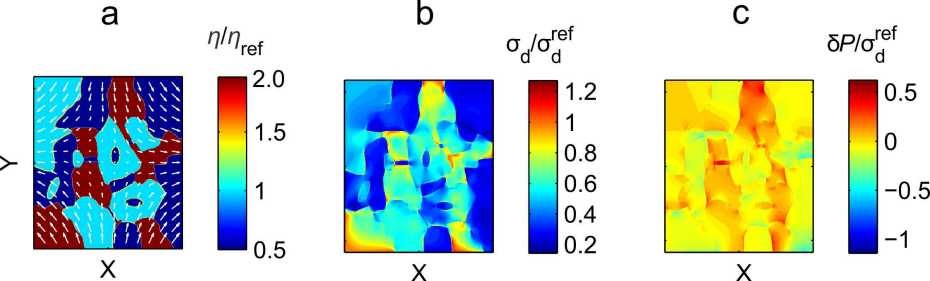
Interestingly, pressure variation can develop in all materials, including rocks, due to deformation. In fact, the topography and variations in rock properties lead to a development of tectonic overpressure (i.e. where the pressure (mean stress) is higher than the lithostatic pressure) or its opposite case, underpressure. Such pressure anomalies are not associated with a particular depth. The deviation does not have to be extreme; over and under pressure can be just few MPa (i.e. much smaller than the uncertainties related to petrological estimates).
Our group has spent last decade developing quantification tools for systems under pressure gradients. It involves an equilibrium thermodynamic formulation for systems under pressure gradients or a coupled model for chemical diffusion and mechanical deformation in analogy to the studies of poroelasticity and thermoelasticity. With the new approaches, we can numerically simulate the effect of chemical diffusion on the development of a pressure gradient across a mineral grain and vice versa. Furthermore, by combining direct stress measurements by Raman spectroscopy and mechanical solutions for stress relaxation in a mineral, we were able to develop a unique method that allows stress relaxation to be calculated directly from natural samples.
More to this topic and the relevant references can be found in papers in the Publications section on this web page.